Differentiation: An introduction to Calculus
This article will be relevant to you if you are studying any of:
- Edexcel IGCSE Maths
- A-level Maths
- BTEC Level 3 Engineering (Module 7)
… or any other course that involves Calculus.
Calculus is a major branch of mathematics consisting of two areas: differentiation and integration. Differentiation involves working with rates of change; integration is the opposite process.
This article introduces you to the idea of differentiation and how to use it with simple algebraic functions. Integration (required for Level 3 courses but not for the IGCSE) isn’t covered here.
The concept of differentiation
Gradients of lines and curves
The gradient of a graph can also be described as the “rate of change of y with respect to x”, because it tells us how quickly the y-value changes compared to the x-value.
A straight line graph has a constant gradient, so it has a constant rate of change throughout its length.
But with a curve, the gradient changes as you move along it.
The gradient of the curve at a point is the same as the gradient of a tangent drawn at that point.

The black curve above is the graph of y = x².
The red line is the tangent to the curve at the point (-1, 1) and the green one is the tangent at the point (2, 4).
You can see that the red tangent has a negative gradient – if you draw a gradient triangle then you can see that the gradient is -2. This tells us that, at the point where x = -1, the gradient of the curve is -2.
But the green tangent has a positive gradient. What is its gradient?
If you work it out, you should find that the gradient is 4. So at the point on the curve where x = 2, the gradient of the curve is 4.
You can estimate the gradient of a graph by drawing a tangent and finding its gradient – you’ve probably done that in science – but because you’re doing it by eye, it’s only an estimate.
Calculus – or, more specifically, the branch of calculus known as differentiation – allows you to calculate the exact gradient of a curve at any point if you know the equation of the curve.
How does differentiation work?
At A-level you’ll need to be able to differentiate from first principles, but for IGCSE you really just need to know how to do the process, not really to understand why it works as it does. Here’s a quick outline of what’s happening.

On the graph above, the darker blue line is the tangent to the curve at point P, and its gradient is the gradient of the curve at that point.
But we need two coordinate pairs to work out a gradient, so we can come up with a rough estimate of the gradient by using a chord that cuts the curve in two places.
You can see that the light green chord is going to give a pretty poor estimate because the points where it crosses the curve are so far apart. But as the second point on the curve is moved closer to P, the chord is getting closer to the tangent so the estimate improves.
Eventually, when the distance between the two points is vanishingly small, the chord becomes indistinguishable from the tangent and so the gradient of the chord is the same as that of the tangent and the curve at that point.
When we differentiate an expression for ![]() , it gives us a new expression called the “first derivative” or
, it gives us a new expression called the “first derivative” or ![]() (pronounced “d y by d x”), and this expression tells us the gradient of the curve as a function of
(pronounced “d y by d x”), and this expression tells us the gradient of the curve as a function of ![]() .
.
![]() means “a little bit of y over a little bit of x” because what we’re effectively doing is finding the gradient of a chord between two points on the curve that are so close together that they are on the point of merging into one, which means that the chord is indistinguishable from the tangent.
means “a little bit of y over a little bit of x” because what we’re effectively doing is finding the gradient of a chord between two points on the curve that are so close together that they are on the point of merging into one, which means that the chord is indistinguishable from the tangent.
If the original function is expressed using ![]() notation then the first derivative is called
notation then the first derivative is called ![]() (“f prime of x”) instead of
(“f prime of x”) instead of ![]() , but it’s still the same thing.
, but it’s still the same thing.
Using differentiation in simple situations
How to differentiate simple expressions
If ![]() then
then ![]()
– i.e. we multiply by the power and decrease the power by 1.
So if ![]() then
then ![]()
What’s ![]() if
if ![]() ?
?
Answer: ![]() .
.
It works in the same way if there’s a coefficient in front of the ![]() :
:
If ![]() then
then ![]()
So if ![]() then
then
![]()
And we can differentiate expressions consisting of terms that are added/subtracted:
If ![]() then
then ![]()
Look at what happens with linear expressions and constants:
![]() can be thought of as
can be thought of as ![]() .
.
What will happen when you apply the same process here?
Answer: ![]()
Notice how ![]() disappears leaving only its coefficient, and a constant disappears altogether.
disappears leaving only its coefficient, and a constant disappears altogether.
Of course, you already know that ![]() is the equation of a straight line graph with a constant gradient of 2, and the graph of
is the equation of a straight line graph with a constant gradient of 2, and the graph of ![]() would be a horizontal line with a gradient of 0, so this result should make sense!
would be a horizontal line with a gradient of 0, so this result should make sense!
Finding the gradient at a given point
To find the gradient of a curve at a particular point, or a particular value of ![]() , all you have to do is substitute the value of
, all you have to do is substitute the value of ![]() into the expression for
into the expression for ![]() .
.
(The y-coordinate is irrelevant when finding the gradient.)
Previously we established that if ![]() then the gradient is given by
then the gradient is given by ![]() .
.
So at the point where ![]() , the gradient is
, the gradient is ![]()
and at the point where ![]() , the gradient is
, the gradient is ![]()
What would the gradient of the curve ![]() be at the point
be at the point ![]() ?
?
Answer: ![]() so the gradient would be
so the gradient would be ![]() .
.
Your turn 1
For each of the functions listed below, find the first derivative ![]() , and hence find the gradients when
, and hence find the gradients when ![]() and when
and when ![]() .
.
Differentiation with negative powers of x
Differentiation works in exactly the same way with negative powers of ![]() – and also with fractional powers, though that’s not covered here (and isn’t required for IGCSE).
– and also with fractional powers, though that’s not covered here (and isn’t required for IGCSE).
For example, if ![]() then
then ![]()
Careful – remember that decreasing -3 by 1 gives you -4, not -2!
Of course, this could also have been given to you as ![]() . If you get an expression in this form then always write it as
. If you get an expression in this form then always write it as ![]() before trying to differentiate it.
before trying to differentiate it.
So if ![]() then that’s the same as
then that’s the same as ![]()
and so ![]()
It’s usually helpful to put it back into fraction form after differentiating, though, as that makes calculations easier. In the above example, that would of course give us ![]() .
.
Factorised expressions
If you’re given a factorised expression then you need to expand it before you differentiate.
(In some cases this isn’t necessary, but that requires techniques that come much later!)
For example, ![]() would have to be expanded to give
would have to be expanded to give ![]() before any differentiation could take place.
before any differentiation could take place.
Finding a point with a given gradient
To identify a point with a given gradient, find the expression for ![]() and put it equal to the gradient specified, then solve to find
and put it equal to the gradient specified, then solve to find ![]() .
.
Example: Find the coordinates of the point on the curve of ![]() where the gradient is 3.
where the gradient is 3.
Solution: ![]() and gradient
and gradient ![]()
So ![]()
Solve to get ![]()
Finally substitute back into the original equation to find ![]() :
:![]()
So the gradient is 3 at the point ![]() .
.
The image below shows the curve with its tangent of gradient 3 at the point ![]() .
.

Your turn 2
For each of the functions below, find (a) ![]() , and (b) the gradient when x = -1. For questions 1 to 3, also find the coordinates of the point(s) on the curve where the gradient is 1.
, and (b) the gradient when x = -1. For questions 1 to 3, also find the coordinates of the point(s) on the curve where the gradient is 1.
You might like to use Desmos to plot the graphs and the tangents at the points you’ve identified, to see how they relate – like in the image above. (For each tangent, you know that the gradient is 1 and you’ve found the coordinates of a point that’s on the line, so it’s easy to work out its equation from that.)
Other uses of differentiation
Differentiation can also be used to find the stationary points on a curve and to determine whether a stationary point is a maximum, a minimum or a point of inflection.
It can be used to identify intervals of increase and decrease in a function (i.e. for which values of x the value of y is going up or down / the gradient is positive or negative).
It can be used in the context of kinematics to move between displacement, velocity and acceleration.
And it can be used to solve optimisation problems in practical situations.
But we’ll leave all those for future articles – I think you deserve a rest now!
If you’ve found this article helpful then please share it with anyone else who you think would benefit (use the social sharing buttons if you like). If you have any suggestions for improvement or other topics that you’d like to see covered, then please comment below or drop me a line using my contact form.
On my sister site at at mathscourses.co.uk you can find – among other things – a great-value suite of courses covering the entire GCSE (and Edexcel IGCSE) Foundation content, and the “Flying Start to A-level Maths” course for those who want to get top grades at GCSE and hit the ground running at A-level – please take a look!
If you’d like to be kept up to date with my new content then please sign up to my mailing list using the form at the bottom of this page, which will also give you access to my collection of free downloads.
Answers:
Your turn 1 answers
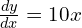 ; gradients 20, -10
; gradients 20, -10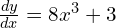 ; gradients 67, -5
; gradients 67, -5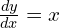 ; gradients 2, -1
; gradients 2, -1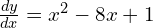 ; gradients -27, 10
; gradients -27, 10
Click here to return to questions
Your turn 2 answers
- (a)
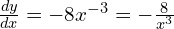 ; (b) gradient = 8; (c)
; (b) gradient = 8; (c) 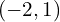
- (a)
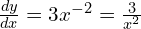 ; (b) gradient = 3; (c)
; (b) gradient = 3; (c) 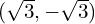 and
and 
- (a)
 ; (b) gradient = 1; (c)
; (b) gradient = 1; (c) 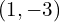 and
and 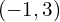
- (a)
 ; (b) gradient = -5
; (b) gradient = -5
